From the ALWS archives: A selection of papers from the International Wittgenstein Symposia in Kirchberg am Wechsel

• Home
• About
• Login
• Search
• Current
• Archives

Table of contents

• Literature

The notion of image (Bild) is a fundamental one in the Tractatus. This notion is immediately introduced in the text after the brief ontological section, because it serves to give an account in logical terms of our relationship with the world. The relationship between fact and image is generally considered as governed by a form of isomorphism. Here I want to maintain that it is not a matter of isomorphism, but instead of homomorphism.
The notion of Bild is introduced with a figurative image:

T. 2.1 Wir machen Uns Bilder der Tatsachen,

or as we find in the ProtoTractatus:

PT. 2.1 Die Tatsachen begreifen wir in Bildern.

In German, as in many modern languages, the notion of Bild has a large variety of meanings, which, however, can usefully be reduced to 2 (+1 the adult stage of insects, which here does not interest us). A representational meaning (1), whereby a certain thing is an image of another if in certain respects it preserves a certain similarity to it: a photograph is an image, a model is an image, and so forth; or in another mathematical meaning (2), the image is the set Y of the values of a function F(X), and at the same time the value y of the function f(x) and is said to be an image of x with respect to F.

Though it may appear pleonastic, we define a function F as a correspondence between two sets X and Y, such that to every element x of X there corresponds only one element y of Y. The set X is referred to as the domain of the function, and the set Y is said is referred to as the co-domain or image of the function F.

Every meaning of the notion of image, both 1 and 2, can be linked to the mathematical meaning of the term, as was done by Lo Piparo (1998), showing that if a certain configuration is an image of something, a function exists that founds the correspondence. Evidently the mathematical meaning of the term does not account for all the characteristics of the image, for example representative immediateness or similarity, but to some extent it delimits its most general possibilities.
In a 1931 colloquy with Waismann, Wittgenstein says (WWK, 185):

I have inherited this concept [of image] on two sides: firstly from the drawn image and secondly from the image of the mathematician, which is already a general concept.

Most scholars interpret the relationship that links the image to the fact represented as a kind of isomorphism. Seeking to give an informal definition of isomorphism, Hofstadter (1979) writes: “a transformation that preserves all the information.” We were saying that the relationship of isomorphism characterizes some one-to-one correspondences such that to every element of the set X there corresponds only one element of the set Y. The correspondence is both surjective and injective. Isomorphism is a one-to-one correspondence between two sets endowed with structures preserving structures of it. Depending on the characteristics of the correspondence, we will define the function as one-to-one if each element of the set Y is the image of only one element of the set X.

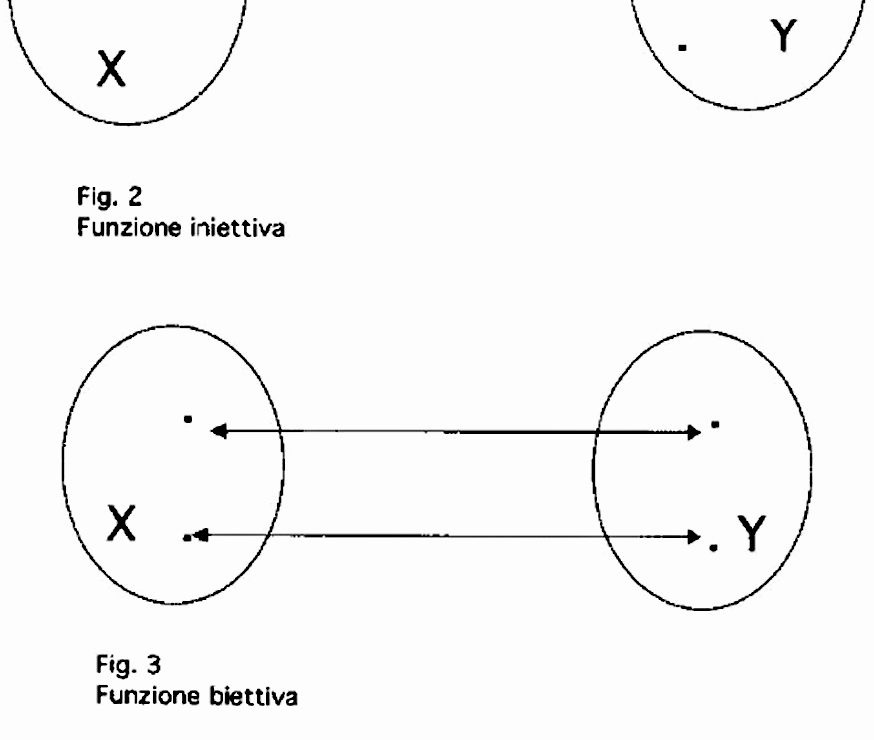
If the two sets are governed by a one-to-one correspondence, then a function exists that we call inverse function, such that to every element y of Y there is made to correspond only one element of X. It is a function through which from the co-domain I can get back to the original set. It is evident that only in the case in which they are in a one-toone correspondence, the sets must have the same number of elements, as can be seen from figures 3. A relationship of structural similarity that preserves properties and relationships, but not biuniqueness, is a relationship of homomorphism. Two structures are said to be homomorphic if, considered two non-empty sets X and Y, respectively domain and co-domain, the elements of the two sets are in such a relationship that if n elements of the set X have a certain relationship with one another, their counterparts in Y will have a corresponding relationship.

Examples of isomorphism are: A musical canon, for example, i.e. a composition in which the same theme is copied and varied in the pitch of the sounds at every entrance of a voice, as happens in Frère Jacques, is an example of isomorphic transformation. Every melodic line of the canon preserves all the information of the previous melodic line. Certainly not a variation. Two chessboards differing in size and material have an isomorphic relationship because the grid of the chessboard is the same and the number of pieces and their function is the same – the rules of chess are the same in both the chessboards.Two isomorphic structures can also be visually less similar; a topological transformation of a space can be governed by a relationship of isomorphism.

The thesis of isomorphism seems, however, at first sight, to be full of textual echoes:

T. 2.13 Den Gegenständen entsprechen im Bilde die Elemente des Bildes.

T. 2.131 Die Elemente des Bildes vertreten im Bild die Gegenstände.

T. 2.15 Daß sich die Elemente des Bildes in bestimmter Art und Weise zu einander verhalten, stellt vor, daß sich die Sachen so zu einander verhalten.

The relationship of isomorphism is certainly compatible with these affirmations, but it appears to be too strong to explain other claims by the philosopher:

T. 4.002 Der Mensch besitzt die Fähigkeit Sprachen zu bauen, womit sich jeder Sinn ausdrücken läßt, ohne eine Ahnung davon zu haben, wie und was jedes Wort bedeutet. — Wie man auch spricht, ohne zu wissen, wie die einzelnen Laute hervorgebracht werden.

Die Umgangssprache ist ein Teil des menschlichen Organismus, und nicht weniger kompliziert als dieser. Es ist menschenunmöglich, die Sprachlogik aus ihr unmittelbar zu entnehmen.
Die Sprache verkleidet den Gedanken. Und zwar so, daß man nach der äußeren Form des Kleides, nicht auf die Form des bekleideten Gedankens schließen kann; weil die äußere Form des Kleides nach ganz anderen Zwecken geBildet ist, als danach, die Form des Körpers erkennen zu lassen. [italics added]

This image is very strong and only apparently repeats Frege’s sartorial famous image (1918, p. 60), which is substantially modified. One author uses the verb kleiden "to wear", the other the verb verkleiden, “to wear, disguise oneself, mask oneself”:

Der an sich unsinnliche Gedanke kleidet sich in das sinnliche Gewand des Satzes und wird uns damit faßbarer.

The most enlightening example of a homomorphic application is that of the projection: a projection preserves the structural interrelations but does not necessarily preserve the equipotentiality. In this case the inverse function does not exist. I cannot always get back from the result of a projection to the original, as can be deduced from the example, where we clearly see the variety of figures that a projection can take on: the projection is compatible with different images, and indeed beginning from the result of the projection we cannot infer the fact projected. For the relationship that links objects to the origin of the projection and the result is a homomorphic relationship and not an isomorphic one: the fact is that there is not necessarily a one-to-one correspondence between the two sets of points that constitute the figures.

Wittgenstein often has recourse to the notion of projection in the Tractatus:

T. 3.11 Wir benützen das sinnlich wahrnehmbare Zeichen (Laut- oder Schriftzeichen etc.) des Satzes als Projektion der möglichen Sachlage.

Die Projektionsmethode ist das Denken des Satz-Sinnes.

T. 3.12 ... Und der Satz ist das Satzzeichen in seiner projektiven Beziehung zur Welt.

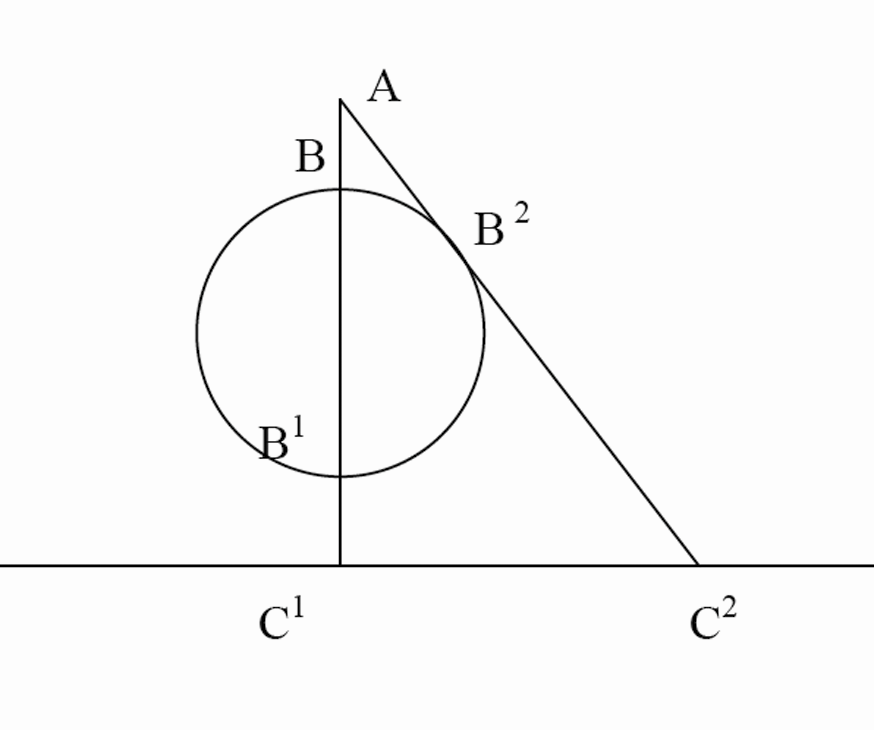
As you can see, despite the point C² is a projection of the B², the point C¹ is the projection of both the points B and B¹.

An indication of the fact that the metaphor of projection in the Tractatus is deliberately used to show the irreducible deformation between the level of facts and that of images comes to us from the 1929 essay Some Remarks on Logical Form (p.30):

Let us imagine two parallel planes, I and II. On plane I figures are drawn, say, ellipses and rectangle of different size and shapes, and it is our task to produce images of these figures on plane II. Then we can imagine two ways, amongst others, of doing this. We can, first, lay down a law of projection [...] and then proceed to project all figures from I into II, according to this law. Or, secondly, we could proceed thus: We lay down the rule that every ellipse on plane I is to appear as a circle in plane II, and every rectangle as a square in II. [...]. We can only gather from them that the original was an ellipse or a rectangle.[...] The case of ordinary language is quite analogous. If the fact of reality are the ellipses and rectangles on plane I the subject-predicate and relational forms correspond to the circles and square on plane II. [italics added]

Though through a later text, but it seems to me that an important characteristic of the relationship of projection identified by Wittgenstein consists in the fact that from what is represented it is not possible to infer what was to be represented if I do not exactly know the method of projection. And also in this case could not exists an inverse function.

In language, in particular, at every level of realization we are faced with different types of deformations and the relationship between the planes that those levels realize is never characterized by biuniqueness and isomorphism, but more often by non-biuniqueness.

The relationship that links written language to oral is a homomorphic relationship and not an isomorphic one; the fact is that in written language there are signs which do not correspond to any sound, like punctuation marks or letters that take on different sounds according to their position: for example in Italian the letter ‘c’ in the word cicca in the first occurrence is an unvoiced pre-palatal affricate and in the second an unvoiced velar plosive, or again there is the letter ‘h’ which in English often does not correspond to any sound, for instance in eight.
(Also non-isomorphic is the relationship between the international phonetic alphabet – though it was conceived as a one-to-one correspondence between sounds and written signs, such that to every sound there would correspond only one letter – and in any spoken language, for example Italian, to each sound there does not correspond a grapheme of the phonetic alphabet. In the previously mentioned case of cicca, to the first occurrence there corresponds /tS/ and to the second /k/, and so there is a double sign /tS/ for a single sound.)
In language isomorphic correspondences do not exist.
The fact is that each plane of language is regulated by autonomous schemes of self-organization that modify the number of elements present.

T 4.014 Die Grammophonplatte, der musikalische Gedanke, die Notenschrift, die Schallwellen, stehen alle in jener abBildenden internen Beziehung zueinander, die zwischen Sprache und Welt besteht.

Ihnen allen ist der logische Bau gemeinsam. (Wie im Märchen die zwei Jünglinge, ihre zwei Pferde und ihre Lilien. Sie sind alle in gewissem Sinne Eins.)

This section takes on a particular role in the economy of the work; the whole section is a long and insistent exemplification of the idea that in order to represent a fact the image has to have in common with it a structure or a logical form, and that this is not immediately recognizable. The same thing happens to a musical theme that lives in the score, as in the performance, or in the record itself, remaining itself each time, although the musical passage in the score contains some notations that the performance cannot reproduce; for example the notational system. And instead it is possible to reconstruct a score beginning from a recording in a disk (this was done, for example, with The Köln Concert (1975) by Keith Jarrett), and it seems that the score reconstructed in this way is quite precise. What a traditional score cannot contain are the metronomic speeds that each performer chooses, though within a certain limit. A performance by Arturo Toscanini and one by Wilhelm Furtwängler are both images of the same score but have different metronomic speeds, so that the performance by the Italian conductor, famous for his accelerated tempos, will have a markedly shorter duration than the one by the German conductor. Performance forces the musician to give a precise value to tempos, as well as to indications relating to intensities (piano and forte), which cannot be written in the score but plays a fundamental role in the interpretation of the piece. Each level of representation has characteristics of its own that make the image irreducible to the fact represented.

But a musical theme does not exist beyond its realization in signs. Conversely, inside the image, the representative modalities of the image itself give rise to objects that present themselves as such in the story there are not horses, children or lilies independently of the image itself, and instead they all constitute and articulate the image together. Indeed, once the image is understood in its fullness, it little matters what the nature of the single constituents of the image is, that is to say whether they are children, horses or lilies; they all are part of the same image, and it is this image in its unity that is understood.

Besides, we have a further sign that homomorphism is the relationship identified by Wittgenstein.

T.4.01 Der Satz ist ein Bild der Wirklichkeit.

Der Satz ist ein Modell der Wirklichkeit, so wie wir sie uns denken.

It is generally accepted as a reference to Hertz, who is the true forerunner of the idea of image expressed in the Tractatus, without necessarily accepting all the observations by Toulmin regarding the idea that Bild should be seen as “Model”; that Wittgenstein is here referring to Hertz is confirmed by proposition 4.04, which expressly quotes the German physicist.

Following this suggestion, in Prinzipien der Mechanik (§418) we find the definition of dynamic models: a material system is called a dynamic model of a second system if the connections of the former can be represented through coordinates such as to satisfy the following conditions:

• 1) the number of coordinates of the first system is equal to the number of coordinates of the second.
• 2) with appropriate rearrangement of the coordinates, the same condition equations hold for both systems.
• 3) with this rearrangement of the coordinates, the expression of the modulus of a displacement coincides for both systems.

In the language of classical physics, system equations are the equations that describe the state of a system through the appropriate coordinates. The coordinates referred to here are size, or degrees of freedom, indispensable for describing a system. A solid cannot be described by a system of two Cartesian axes, which instead perfectly describes a plane figure. A geometrical system requires the three dimensions of the plane, while in order to describe a physical system it may be necessary to consider temperature, gravitational fields or pressure, as in the case in which a system of fluids is considered. Physical models (unlike mathematical ones) are generally governed by a homomorphic relationship (the number of particles in the model is very small in comparison to the number of molecules in a fluid).

The logical multiplicity which Wittgenstein refers to is a concept closely linked to that of the degrees of freedom of a physical system. Indeed, logical multiplicity is given by the characteristics pertinent to describing a system. A model can describe a certain system if it shares its logical multiplicity. Therefore what is required is homomorphism and not isomorphism.

Refbacks

• There are currently no refbacks.